Javascript required
Skip to content Skip to sidebar Skip to footer
Home / Find the Solution to the Differential Equation Db/dt

Find the Solution to the Differential Equation Db/dt

7.E: Differential Equations (Exercises)

  1. Last updated
  2. Save as PDF
  • Page ID
    5402
    • ac-header.png
    • Professors (Mathmatics) at Grand Valley State University
    • Publisher: ScholarWorks @Grand Valley State University

    7.1: An Introduction to Differential Equations

    Q7.1.1

    Suppose that T(t) represents the temperature of a cup of coffee set out in a room, where T is expressed in degrees Fahrenheit and t in minutes. A physical principle known as Newton's Law of Cooling tells us that dT dt = − 1 15 T + 5.

    1. Supposes that T(0) = 105. What does the differential equation give us for the value of dT dt |T=105? Explain in a complete sentence the meaning of these two facts.
    2. Is T increasing or decreasing at t = 0?
    3. What is the approximate temperature at t = 1?
    4. On the graph below, make a plot of dT/dt as a function of T.

    alt

    1. For which values of T does T increase? For which values of T does T decrease? 396 7.1. AN INTRODUCTION TO DIFFERENTIAL EQUATIONS
    2. What do you think is the temperature of the room? Explain your thinking.
    3. Verify that T(t) = 75 + 30e −t/15 is the solution to the differential equation with initial value T(0) = 105. What happens to this solution after a long time?

    Q7.1.2

    Suppose that the population of a particular species is described by the function P(t), where P is expressed in millions. Suppose further that the population's rate of change is governed by the differential equation dP dt = f (P) where f (P) is the function graphed below.

    alt

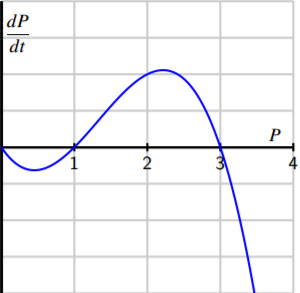
    1. For which values of the population P does the population increase?
    2. For which values of the population P does the population decrease?
    3. If P(0) = 3, how will the population change in time?
    4. If the initial population satisfies 0 < P(0) < 1, what will happen to the population after a very long time?
    5. If the initial population satisfies 1 < P(0) < 3, what will happen to the population after a very long time?
    6. If the initial population satisfies 3 < P(0), what will happen to the population after a very long time?
    7. This model for a population's growth is sometimes called "growth with a threshold." Explain why this is an appropriate name.

    Q7.1.3

    In this problem, we test further what it means for a function to be a solution to a given differential equation. 7.1. AN INTRODUCTION TO DIFFERENTIAL EQUATIONS 397

    1. Consider the differential equation dy dt = y − t. Determine whether the following functions are solutions to the given differential equation.
      1. (i) y(t) = t + 1 + 2e t
      2. (ii) y(t) = t + 1
      3. (iii) y(t) = t + 2
    2. When you weigh bananas in a scale at the grocery store, the height h of the bananas is described by the differential equation d 2h dt2 = −k h where k is the spring constant, a constant that depends on the properties of the spring in the scale. After you put the bananas in the scale, you (cleverly) observe that the height of the bananas is given by h(t) = 4 sin(3t). What is the value of the spring constant?

    7.2: Qualitative Behavior of Solutions to DE's

    Q7.2.1

    Consider the differential equation dy dt = t − y.

    alt

    1. Sketch a slope field on the plot below:
    2. Sketch the solutions whose initial values are y(0) = −4, −3, . . ., 4.
    3. What do your sketches suggest is the solution whose initial value is y(0) = −1? Verify that this is indeed the solution to this initial value problem.
    4. By considering the differential equation and the graphs you have sketched, what is the relationship between t and y at a point where a solution has a local minimum?

    Q7.2.2

    Consider the situation from problem 2 of Section 7.1: Suppose that the population of a particular species is described by the function P(t), where P is expressed in millions. Suppose further that the population's rate of change is governed by the differential equation dP dt = f (P) where f (P) is the function graphed below.

    alt

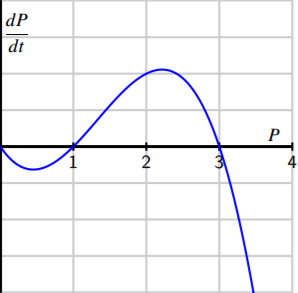
    1. Sketch a slope field for this differential equation. You do not have enough information to determine the actual slopes, but you should have enough information to determine where slopes are positive, negative, zero, large, or small, and hence determine the qualitative behavior of solutions. 7.2. QUALITATIVE BEHAVIOR OF SolutionS TO DES 407
    2. Sketch some solutions to this differential equation when the initial population P(0) > 0.
    3. Identify any equilibrium solutions to the differential equation and classify them as stable or unstable.
    4. If P(0) > 1, what is the eventual fate of the species?
    5. if P(0) < 1, what is the eventual fate of the species?
    6. Remember that we referred to this model for population growth as "growth with a threshold." Explain why this characterization makes sense by considering solutions whose inital value is close to 1.

    Q7.2.3

    The population of a species of fish in a lake is P(t) where P is measured in thousands of fish and t is measured in months. The growth of the population is described by the differential equation dP dt = f (P) = P(6 − P).

    1. Sketch a graph of f (P) = P(6 − P) and use it to determine the equilibrium solutions and whether they are stable or unstable. Write a complete sentence that describes the long-term behavior of the fish population.
    2. Suppose now that the owners of the lake allow fishers to remove 1000 fish from the lake every month (remember that P(t) is measured in thousands of fish). Modify the differential equation to take this into account. Sketch the new graph of dP/dt versus P. Determine the new equilibrium solutions and decide whether they are stable or unstable.
    3. Given the situation in part (b), give a description of the long-term behavior of the fish population.
    4. Suppose that fishermen remove h thousand fish per month. How is the differential equation modified?
    5. What is the largest number of fish that can be removed per month without eliminating the fish population? If fish are removed at this maximum rate, what is the eventual population of fish?

    Q7.2.4

    Let y(t) be the number of thousands of mice that live on a farm; assume time t is measured in years.2

    1. The population of the mice grows at a yearly rate that is twenty times the number of mice. Express this as a differential equation. 2This problem is based on an ecological analysis presented in a research paper by C.S. Hollings: The Components of Predation as Revealed by a Study of Small Mammal Predation of the European Pine Sawfly, Canadian Entomology 91: 283-320.
    2. At some point, the farmer brings C cats to the farm. The number of mice that the cats can eat in a year is M(y) = C y 2 + y thousand mice per year. Explain how this modifies the differential equation that you found in part a).
    3. Sketch a graph of the function M(y) for a single cat C = 1 and explain its features by looking, for instance, at the behavior of M(y) when y is small and when y is large.
    4. Suppose that C = 1. Find the equilibrium solutions and determine whether they are stable or unstable. Use this to explain the long-term behavior of the mice population depending on the initial population of the mice.
    5. Suppose that C = 60. Find the equilibrium solutions and determine whether they are stable or unstable. Use this to explain the long-term behavior of the mice population depending on the initial population of the mice.
    6. What is the smallest number of cats you would need to keep the mice population from growing arbitrarily large?

    7.3: Euler's Method

    Q7.3.1

    Newton's Law of Cooling says that the rate at which an object, such as a cup of coffee, cools is proportional to the difference in the object's temperature and room temperature. If T(t) is the object's temperature and Tr is room temperature, this law is expressed at dT dt = −k(T − Tr ), where k is a constant of proportionality. In this problem, temperature is measured in degrees Fahrenheit and time in minutes.

    1. Two calculus students, Alice and Bob, enter a 70◦ classroom at the same time. Each has a cup of coffee that is 100◦ . The differential equation for Alice has a constant of proportionality k = 0.5, while the constant of proportionality for Bob is k = 0.1. What is the initial rate of change for Alice's coffee? What is the initial rate of change for Bob's coffee?
    2. What feature of Alice's and Bob's cups of coffee could explain this difference?
    3. As the heating unit turns on and off in the room, the temperature in the room is Tr = 70 + 10 sin t. Implement Euler's method with a step size of ∆t = 0.1 to approximate the temperature of Alice's coffee over the time interval 0 ≤ t ≤ 50. This will most easily be performed using a spreadsheet such as Excel. Graph the temperature of her coffee and room temperature over this interval.
    4. In the same way, implement Euler's method to approximate the temperature of Bob's coffee over the same time interval. Graph the temperature of his coffee and room temperature over the interval.
    5. Explain the similarities and differences that you see in the behavior of Alice's and Bob's cups of coffee.

    Q7.3.2

    We have seen that the error in approximating the solution to an initial value problem is proportional to ∆t. That is, if E∆t is the Euler's method approximation to the solution to an initial value problem at t, then y(t) − E∆t ≈ K∆t for some constant of proportionality K. In this problem, we will see how to use this fact to improve our estimates, using an idea called accelerated convergence.

    1. We will create a new approximation by assuming the error is exactly proportional to ∆t, according to the formula y(t) − E∆t = K∆t. Using our earlier results from the initial value problem dy/dt = y and y(0) = 1 with ∆t = 0.2 and ∆t = 0.1, we have y(1) − 2.4883 = 0.2K y(1) − 2.5937 = 0.1K. This is a system of two linear equations in the unknowns y(1) and K. Solve this system to find a new approximation for y(1). (You may remember that the exact value is y(1) = e = 2.71828 . . . .)
    2. Use the other data, E0.05 = 2.6533 and E0.025 = 2.6851 to do similar work as in to obtain another approximation. Which gives the better approximation? Why do you think this is?
    3. Let's now study the initial value problem dy dt = t − y, y(0) = 0. Approximate y(0.3) by applying Euler's method to find approximations E0.1 and E0.05. Now use the idea of accelerated convergence to obtain a better approximation. (For the sake of comparison, you want to note that the actual value is y(0.3) = 0.0408.)

    Q7.3.3

    In this problem, we'll modify Euler's method to obtain better approximations to solutions of initial value problems. This method is called the Improved Euler's method. In Euler's method, we walk across an interval of width ∆t using the slope obtained from the differential equation at the left endpoint of the interval. Of course, the slope of the solution will most likely change over this interval. We can improve our approximation by trying to incorporate the change in the slope over the interval. Let's again consider the initial value problem dy/dt = y and y(0) = 1, which we will approximate using steps of width ∆t = 0.2. Our first interval is therefore 0 ≤ t ≤ 0.2. At t = 0, the differential equation tells us that the slope is 1, and the approximation we obtain from Euler's method is that y(0.2) ≈ y1 = 1 + 1(0.2) = 1.2. This gives us some idea for how the slope has changed over the interval 0 ≤ t ≤ 0.2. We know the slope at t = 0 is 1, while the slope at t = 0.2 is 1.2, trusting in the Euler's method approximation. We will therefore refine our estimate of the initial slope to be the average of these two slopes; that is, we will estimate the slope to be (1+1.2)/2 = 1.1. This gives the new approximation y(1) = y1 = 1 + 1.1(0.2) = 1.22. The first few steps look like this:

    alt

    1. Continue with this method to obtain an approximation for y(1) = e.
    2. Repeat this method with ∆t = 0.1 to obtain a better approximation for y(1).
    3. We saw that the error in Euler's method is proportional to ∆t. Using your results from parts and (b), what power of ∆t appears to be proportional to the error in the Improved Euler's Method?

    7.4: Separable Differential Equations

    Q7.4.1

    The mass of a radioactive sample decays at a rate that is proportional to its mass.

    1. Express this fact as a differential equation for the mass M(t) using k for the constant of proportionality.
    2. If the initial mass is M0, find an expression for the mass M(t).
    3. The half-life of the sample is the amount of time required for half of the mass to decay. Knowing that the half-life of Carbon-14 is 5730 years, find the value of k for a sample of Carbon-14.
    4. How long does it take for a sample of Carbon-14 to be reduced to one-quarter its original mass?
    5. Carbon-14 naturally occurs in our environment; any living organism takes in Carbon-14 when it eats and breathes. Upon dying, however, the organism no longer takes in Carbon-14. Suppose that you find remnants of a pre-historic firepit. By analyzing the charred wood in the pit, you determine that the amount of Carbon-14 is only 30% of the amount in living trees. Estimate the age of the firepit.5

    Q7.4.2

    Consider the initial value problem dy dt = − t y , y(0) = 8

    1. Find the solution of the initial value problem and sketch its graph.
    2. For what values of t is the solution defined?
    3. What is the value of y at the last time that the solution is defined?
    4. By looking at the differential equation, explain why we should not expect to find solutions with the value of y you noted in (c).

    Q7.4.3

    Suppose that a cylindrical water tank with a hole in the bottom is filled with water. The water, of course, will leak out and the height of the water will decrease. Let h(t) denote the height of the water. A physical principle called Torricelli's Law implies that the height decreases at a rate proportional to the square root of the height.

    1. Express this fact using k as the constant of proportionality.
    2. Suppose you have two tanks, one with k = −1 and another with k = −10. What physical differences would you expect to find? 5This approach is the basic idea behind radiocarbon dating.
    3. Suppose you have a tank for which the height decreases at 20 inches per minute when the water is filled to a depth of 100 inches. Find the value of k.
    4. Solve the initial value problem for the tank in part (c), and graph the solution you determine.
    5. How long does it take for the water to run out of the tank?
    6. Is the solution that you found valid for all time t? If so, explain how you know this. If not, explain why not.

    Q7.4.4

    The Gompertz equation is a model that is used to describe the growth of certain populations. Suppose that P(t) is the population of some organism and that dP dt = −P ln P 3 ! = −P(ln P − ln 3).

    1. Sketch a slope field for P(t) over the range 0 ≤ P ≤ 6.
    2. Identify any equilibrium solutions and determine whether they are stable or unstable.
    3. Find the population P(t) assuming that P(0) = 1 and sketch its graph. What happens to P(t) after a very long time?
    4. Find the population P(t) assuming that P(0) = 6 and sketch its graph. What happens to P(t) after a very long time?
    5. Verify that the long-term behavior of your solutions agrees with what you predicted by looking at the slope field.

    7.5: Modeling with Differential Equations

    Q7.5.1

    Congratulations, you just won the lottery! In one option presented to you, you will be paid one million dollars a year for the next 25 years. You can deposit this money in an account that will earn 5% each year.

    1. Set up a differential equation that describes the rate of change in the amount of money in the account. Two factors cause the amount to grow—first, you are depositing one millon dollars per year and second, you are earning 5% interest.
    2. If there is no amount of money in the account when you open it, how much money will you have in the account after 25 years?
    3. The second option presented to you is to take a lump sum of 10 million dollars, which you will deposit into a similar account. How much money will you have in that account after 25 years?
    4. Do you prefer the first or second option? Explain your thinking.
    5. At what time does the amount of money in the account under the first option overtake the amount of money in the account under the second option?

    Q7.5.2

    When a skydiver jumps from a plane, gravity causes her downward velocity to increase at the rate of g ≈ 9.8 meters per second squared. At the same time, wind resistance causes her velocity to decrease at a rate proportional to the velocity.

    1. Using k to represent the constant of proportionality, write a differential equation that describes the rate of change of the skydiver's velocity.
    2. Find any equilibrium solutions and decide whether they are stable or unstable. Your result should depend on k.
    3. Suppose that the initial velocity is zero. Find the velocity v(t).
    4. A typical terminal velocity for a skydiver falling face down is 54 meters per second. What is the value of k for this skydiver?
    5. How long does it take to reach 50% of the terminal velocity?

    Q7.5.3

    During the first few years of life, the rate at which a baby gains weight is proportional to the reciprocal of its weight.

    1. Express this fact as a differential equation.
    2. Suppose that a baby weighs 8 pounds at birth and 9 pounds one month later. How much will he weigh at one year?
    3. Do you think this is a realistic model for a long time?

    Q7.5.4

    Suppose that you have a water tank that holds 100 gallons of water. A briny solution, which contains 20 grams of salt per gallon, enters the tank at the rate of 3 gallons per minute. At the same time, the solution is well mixed, and water is pumped out of the tank at the rate of 3 gallons per minute.

    1. Since 3 gallons enters the tank every minute and 3 gallons leaves every minute, what can you conclude about the volume of water in the tank.
    2. How many grams of salt enters the tank every minute?
    3. Suppose that S(t) denotes the number of grams of salt in the tank in minute t. How many grams are there in each gallon in minute t?
    4. Since water leaves the tank at 3 gallons per minute, how many grams of salt leave the tank each minute?
    5. Write a differential equation that expresses the total rate of change of S. Identify any equilibrium solutions and determine whether they are stable or unstable.
    6. Suppose that there is initially no salt in the tank. Find the amount of salt S(t) in minute t.
    7. What happens to S(t) after a very long time? Explain how you could have predicted this only knowing how much salt there is in each gallon of the briny solution that enters the tank.

    7.6: Population Growth and the Logistic Equation

    Q7.6.1

    The logistic equation may be used to model how a rumor spreads through a group of people. Suppose that p(t) is the fraction of people that have heard the rumor on day t. The equation dp dt = 0.2p(1 − p) describes how p changes. Suppose initially that one-tenth of the people have heard the rumor; that is, p(0) = 0.1.

    1. What happens to p(t) after a very long time?
    2. Determine a formula for the function p(t).
    3. At what time is p changing most rapidly?
    4. How long does it take before 80% of the people have heard the rumor?

    Q7.6.2

    Suppose that b(t) measures the number of bacteria living in a colony in a Petri dish, where b is measured in thousands and t is measured in days. One day, you measure that there are 6,000 bacteria and the per capita growth rate is 3. A few days later, you measure that there are 9,000 bacteria and the per capita growth rate is 2.

    1. Assume that the per capita growth rate db/dt b is a linear function of b. Use the measurements to find this function and write a logistic equation to describe db dt .
    2. What is the carrying capacity for the bacteria?
    3. At what population is the number of bacteria increasing most rapidly?
    4. If there are initially 1,000 bacteria, how long will it take to reach 80% of the carrying capacity?

    Q7.6.3

    Suppose that the population of a species of fish is controlled by the logistic equation dP dt = 0.1P(10 − P), where P is measured in thousands of fish and t is measured in years.

    1. What is the carrying capacity of this population?
    2. Suppose that a long time has passed and that the fish population is stable at the carrying capacity. At this time, humans begin harvesting 20% of the fish every year. Modify the differential equation by adding a term to incorporate the harvesting of fish.
    3. What is the new carrying capacity?
    4. What will the fish population be one year after the harvesting begins?
    5. How long will it take for the population to be within 10% of the carrying capacity?

    Find the Solution to the Differential Equation Db/dt

    Source: https://math.libretexts.org/Bookshelves/Calculus/Book:_Active_Calculus_%28Boelkins_et_al%29/07:_Differential_Equations/7.E:_Differential_Equations_%28Exercises%29